Lines and Angles
Exercise 5.2
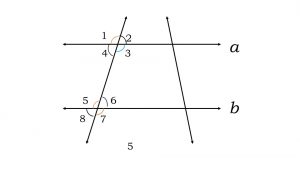
1). State the property that is used in each of the following statements?
(i) If a || b, then ∠1 = ∠5.
(ii) If ∠4 = ∠6, then a || b.
(iii) If ∠4 + ∠5 = 180°, then a || b.
Answer:
(i) If a || b, then ∠1 = ∠5.
Corresponding angles property.
(ii) If ∠4 = ∠6, then a || b.
The converse of alternate angles property.
(iii) If ∠4 + ∠5 = 180°, then a || b.
Interior angles property.
2). In the adjoining figure, identify
(i) the pairs of corresponding angles.
(ii) the pairs of alternate interior angles.
(iii) the pairs of interior angles on the same side of the transversal.
(iv) the vertically opposite angles.

Answer:
(i) the pairs of corresponding angles.
∠1 and ∠5, ∠2 and ∠6, ∠3 and ∠7 and ∠4 and ∠8
(ii) the pairs of alternate interior angles.
∠4 and ∠6 and ∠3 and ∠5
(iii) the pairs of interior angles on the same side of the transversal.
∠3 and ∠6 and ∠4 and ∠5
(iv) the vertically opposite angles.
∠1 and ∠3 and ∠2 and ∠4 ∠6 and ∠7 and ∠5 and ∠8
3). In the adjoining figure, p || q. Find the unknown angles.

1250 and ∠e are forming linear pair
1250 + ∠e = 180
∠e = 180 – 125
∠e = 550
∠e and ∠f are vertically opposite angles
∠e = ∠f
But ∠e = 550
∠f = 550
1250 and ∠d are pair of corresponding angles
∠d = 1250
∠d and ∠b are vertically opposite angles
∠d = ∠b
But ∠d = 1250
∠b = 1250
∠d and ∠c are forming linear pair
∠d + ∠c = 180
But ∠d + = 125
∠c = 180 – 125
∠c = 550
∠a and ∠c are vertically opposite angles
∠a = ∠c
But ∠c = 550
∠a = 550
4). Find the value of x in each of the following figures if l || m.
(i)  (ii)
(ii) 
Solution:
(i) let y be the vertically opposite angle of 1100
y = 1100 (vertically opposite angles are congruent)
l || m and t is transversal
1100 + x0 = 180 (interior angles are supplementary)
x0 =180 – 110
x0 =700
(ii) l || m and a is transversal
x = 1000 (corresponding angles are congruent)
5). In the given figure, the arms of two angles are parallel. If ∠ABC = 70º, then find
(i) ∠DGC
(ii) ∠DEF

Given: ∠ABC = 70º
AB ||DE and BC || EF
Since AB ||DE and BC is transversal
∠ABC and ∠DGC are forming a pair of corresponding angles
∠ABC = ∠DGC
But ∠DGC = 700
∠DGC = 700
Since BC ||EF and DE is transversal
∠DEF and ∠DGC are forming a pair of corresponding angles
∠DEF = ∠DGC
But ∠DGC = 700
∠DEF = 700
6). In the given figures below, decide whether l is parallel to m.
i). 
1260 + 440 = 170
1260 + 440 ≠180
The Sum of interior angles is not equal to 180.
By interior angles test, l is not parallel to m
ii). 
let x be the vertically opposite angle of 750
x = 750 (vertically opposite angles are congruent)
750 + 750 = 150
750 + 750 ≠180
The Sum of interior angles is not equal to 180
By interior angles test, l is not parallel to m
iii). 
let x be the vertically opposite angle of 570
x = 570 (vertically opposite angles are congruent)
570 + 1230 = 180
The Sum of interior angles is equal to 180
By interior angles test, l is parallel to m
iv). 
let x be the vertically opposite angle of 720
x = 720 (vertically opposite angles are congruent)
720 + 980 = 170
750 + 750 ≠180
The Sum of interior angles is not equal to 180
By interior angles test, l is not parallel to m
Click here for the solutions of
![]()
