Squares And Square Roots
Exercise 5.3
1). What could be the possible ‘one’s’ digits of the square root of each of the following
numbers?
(i) 9801 (ii) 99856
(iii) 998001 (iv) 657666025
Solution:
(i) 9801
The possible one’s digit of the square root of 9801 may be 1 or 9. (ii) 99856
The possible one’s digit of the square root of 99856 may be 4 or 6.
(iii) 998001
The possible one’s digit of the square root of 998001 may be 1 or 9.
(iv) 657666025
The possible one’s digit of the square root of 657666025 is 5.
2). Without doing any calculation, find the numbers which are surely not perfect squares.
(i) 153 (ii) 257
(iii) 408 (iv) 441
Solution:
(i) 153
We know that numbers ending with 2, 3, 7 and 8 are not perfect squares.
Therefore 153 is not a perfect square.
(ii) 257
We know that numbers ending with 2, 3, 7 and 8 are not perfect squares.
Therefore 257 is not a perfect square.
(iii) 408
We know that numbers ending with 2, 3, 7 and 8 are not perfect squares.
Therefore 408 is not a perfect square.
(iv) 441
441 is a perfect square.
3). Find the square roots of 100 and 169 by the method of repeated subtraction.
(i) 100
100 – 1 = 99
99 – 3 = 96
96 – 5 = 91
91 – 7 = 84
84 – 9 = 75
75 – 11 = 64
64 – 13 = 51
51 – 15 = 36
36 – 17 = 19
19 – 19 = 0
In the tenth step we got 0 therefore square root of 100 is 10.
(ii) 169
169 – 1 = 168
168 – 3 = 165
165 – 5 = 160
160 – 7 = 153
153 – 9 = 144
144 – 11 = 133
133 – 13 = 120
120 – 15 = 105
105 – 17 = 98
98 – 19 = 79
79 – 21 = 58
58 – 23 = 25
25 – 25 = 0
In the thirteenth step we got 0 therefore square root of 169 is 13.
4). Find the square roots of the following numbers by the Prime Factorisation Method.
(i) 729 (ii) 400
(iii) 1764 (iv) 4096
(v) 7744 (vi) 9604
(vii) 5929 (viii) 9216
(ix) 529 (x) 8100
Solution:
(i) 729

729 = 3 X 3 X 3 X 3 X 3 X 3
= 3 X 3 X 3 X 3 X 3 X 3
= 32 X 32 X 32
√729 = 3 X 3 X 3 = 27
(ii) 400

400 = 2 X 2 X 2 X 2 X 5 X 5
= 2 X 2 X 2 X 2 X 5 X 5
= 22 X 22 X 52
√400 = 2 X 2 X 5 = 20
(iii) 1764
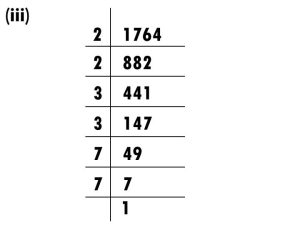
1764 = 2 X 2 X 3 X 3 X 7 X 7
= 2 X 2 X 3 X 3 X 7 X 7
= 22 X 32 X 72
√1764 = 2 X 3 X 7 = 42
(iv) 4096
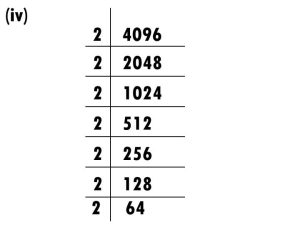
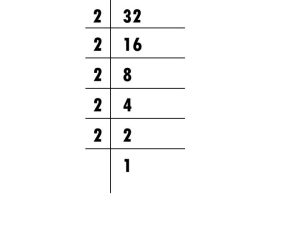
4096 = 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2
= 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2
= 22 X 22 X 22 X 22 X 22 X 22
√4096 = 2 X 2 X 2 X 2 X 2 X 2 = 64
(v) 7744

7744 = 2 X 2 X 2 X 2 X 2 X 2 X 11 X 11
= 2 X 2 X 2 X 2 X 2 X 2 X 11 X 11
= 22 X 22 X 22 X 112
√7744 = 2 X 2 X 2 X 11 = 88
(vi) 9604
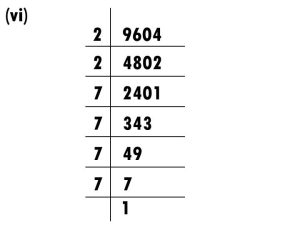
9604 = 2 X 2 X 7 X 7 X 7 X 7
= 2 X 2 X 7 X 7 X 7 X 7
= 22 X 72 X 72
√9604 = 2 X 7 X 7 = 98
(vii) 5929
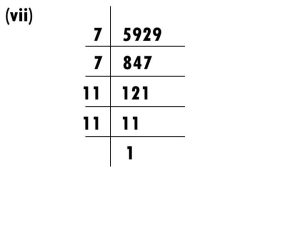
5929 = 7 X 7 X 11 X 11
= 7 X 7 X 11 X 11
= 72 X 112
√5929 = 7 X 11 = 77
(viii) 9216


9216 = 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 3 X 3
= 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 3 X 3
= 22 X 22 X 22 X 22 X 22 X 32
√4096 = 2 X 2 X 2 X 2 X 2 X 3 = 96
(ix) 529

529 = 23 X 23
= 23 X 23
= 232
√529 = 23
(x) 8100

8100 = 2 X 2 X 3 X 3 X 3 X 3 X 5 X 5
= 2 X 2 X 3 X 3 X 3 X 3 X 5 X 5
= 22 X 32 X 32 X 52
√8100 = 2 X 3 X 3 X 5 = 90
5). For each of the following numbers, find the smallest whole number by which it should be multiplied so as to get a perfect square number. Also find the square root of the square number so obtained.
(i) 252 (ii) 180
(iii) 1008 (iv) 2028
(v) 1458 (vi) 768
Solution:
(i) 252

Prime factors of 252 = 2 X 2 X 3 X 3 X 7
Here 7 has no pair
7 is the smallest whole number by which 252 is multiplied to get a perfect square number.
New square number = 252 X 7 = 1764
√1764 = 2 X 3 X 7 = 42
(ii) 180

Prime factors of 180 = 2 X 2 X 3 X 3 X 5
Here 5 has no pair
5 is the smallest whole number by which 180 is multiplied to get a perfect square number.
New square number = 180 X 5 = 900
√900 = 2 X 3 X 5 = 30
(iii) 1008
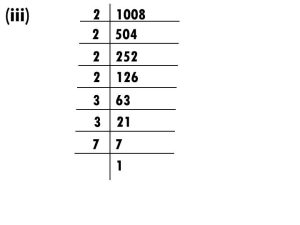
Prime factors of 1008 = 2 X 2 X 2 X 2 X 3 X 3 X 7
Here 7 has no pair
7 is the smallest whole number by which 1008 is multiplied to get a perfect square number.
New square number = 1008 X 7 = 7056
√7056 = 2 X 2 X 3 X 7 = 84
(iv) 2028

Prime factors of 2028 = 2 X 2 X 3 X 13 X 13
Here 3 has no pair
3 is the smallest whole number by which 2028 is multiplied to get a perfect square number.
New square number = 2028 X 3 = 6084
√6084 = 2 X 3 X 13 = 78
(v) 1458
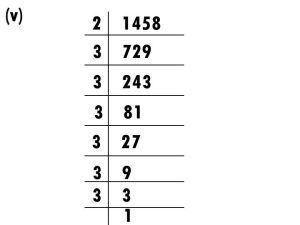
Prime factors of 1458 = 2 X 3 X 3 X 3 X 3 X 3 X 3
Here 2 has no pair
2 is the smallest whole number by which 1458 is multiplied to get a perfect square number.
New square number = 1458 X 2 = 2916
√2916 = 2 X 3 X 3 X 3 = 54
(vi) 768

Prime factors of 768 = 2 X 2 X 2 X 2 X 2 X 2 X 2 X 2 X 3
Here 3 has no pair
3 is the smallest whole number by which 768 is multiplied to get a perfect square number.
New square number = 768 X 3 = 2304
√2304 = 2 X 2 X 2 X 2 X 3 = 48
6). For each of the following numbers, find the smallest whole number by which it should be divided so as to get a perfect square. Also find the square root of the square number so obtained.
(i) 252 (ii) 2925
(iii) 396 (iv) 2645
(v) 2800 (vi) 1620
Solution:
(i) 252

Prime factors of 252 = 2 X 2 X 3 X 3 X 7
Here 7 has no pair
7 is the smallest whole number by which 252 is divided to get a perfect square number.
New square number = 252 ÷ 7 = 36
√36 = 6
(ii) 2925

Prime factors of 2925 = 3 X 3 X 5 X 5 X 13
Here 13 has no pair
13 is the smallest whole number by which 2925 is divided to get a perfect square number.
New square number = 2925 ÷ 13 = 225
√225 = 15
(iii) 396
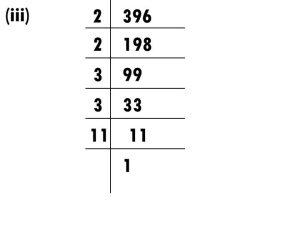
Prime factors of 396 = 2 X 2 X 3 X 3 X 11
Here 11 has no pair
11 is the smallest whole number by which 396 is divided to get a perfect square number.
New square number = 396 ÷ 11 = 36
√36 = 6
(iv) 2645

Prime factors of 2645 = 5 X 23 X 23
Here 5 has no pair
5 is the smallest whole number by which 2645 is divided to get a perfect square number.
New square number = 2645 ÷ 5 = 529
√529 = 23
(v) 2800
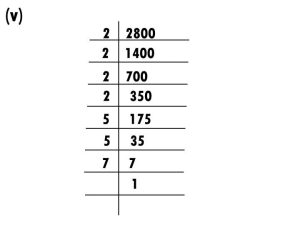
Prime factors of 2800 = 2 X 2 X 2 X 2 X 5 X 5 X 7
Here 7 has no pair
7 is the smallest whole number by which 2800 is divided to get a perfect square number.
New square number = 2800 ÷ 7 = 400
√400 = 20
(vi) 1620
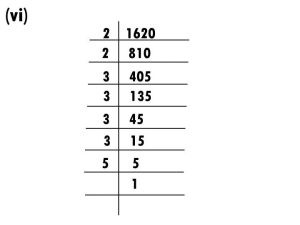
Prime factors of 1620 = 2 X 2 X 3 X 3 X 3 X 3 X 5
Here 5 has no pair
5 is the smallest whole number by which 1620 is divided to get a perfect square number.
New square number = 1620 ÷ 5 = 324
√324 = 18
7). The students of Class VIII of a school donated ₹ 2401 in all, for Prime Minister’s National Relief Fund. Each student donated as many rupees as the number of students in the class. Find the number of students in the class.
Amount donated = 2401
Number of students = amount donated by each student

Number of students = √2401
= √(7 X 7 X 7 X 7)
= √(72 X 72)
= 7 X 7
= 49
Ans: number of students = 49
8). 2025 plants are to be planted in a garden in such a way that each row contains as many plants as the number of rows. Find the number of rows and the number of plants in each row.
Number of plants = 2025
Number of plants in a row = number of rows
Number of plants in each row = √2025
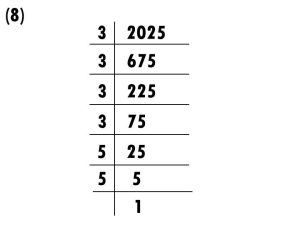
= √(3 X 3 X 3 X 3 X 5 X 5)
= √(32 X 32 X 52)
= 3 X 3 X 5
= 45
Ans: number of plants in each row = 45
9). Find the smallest square number that is divisible by each of the numbers 4, 9 and 10.
Smallest number divisible by each of the numbers 4, 9 and 10 is
LCM of 4 , 9 , 10 = 180
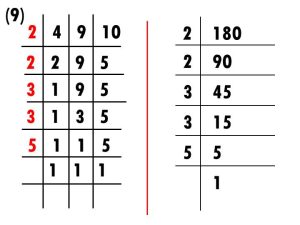
Prime factors of 180 = 2 X 2 X 3 X 3 X 5
Here, 5 has no pair.
The required smallest square number = 180 X 5 = 900
10). Find the smallest square number that is divisible by each of the numbers 8, 15 and 20.
Smallest number divisible by each of the numbers 8, 15 and 20 is
LCM of 8, 15 and 20 = 120

Prime factors of 120 = 2 X 2 X 2 X 3 X 5
Here, 2 X 3 X 5 has no pair.
The required smallest square number = 120 X 30 = 3600
Click here for the solutions of Std 8 Maths
1). Rational Numbers
2). Linear Equations in One Variable
3). Understanding Quadrilaterals
4). Data Handling
5). Squares and Square Roots
![]()